CHAPTER 5 Bound States: Simple Cases Instructor Solutions Manual for Harris, Modern Physics, Second Edition

Document Content and Description Below

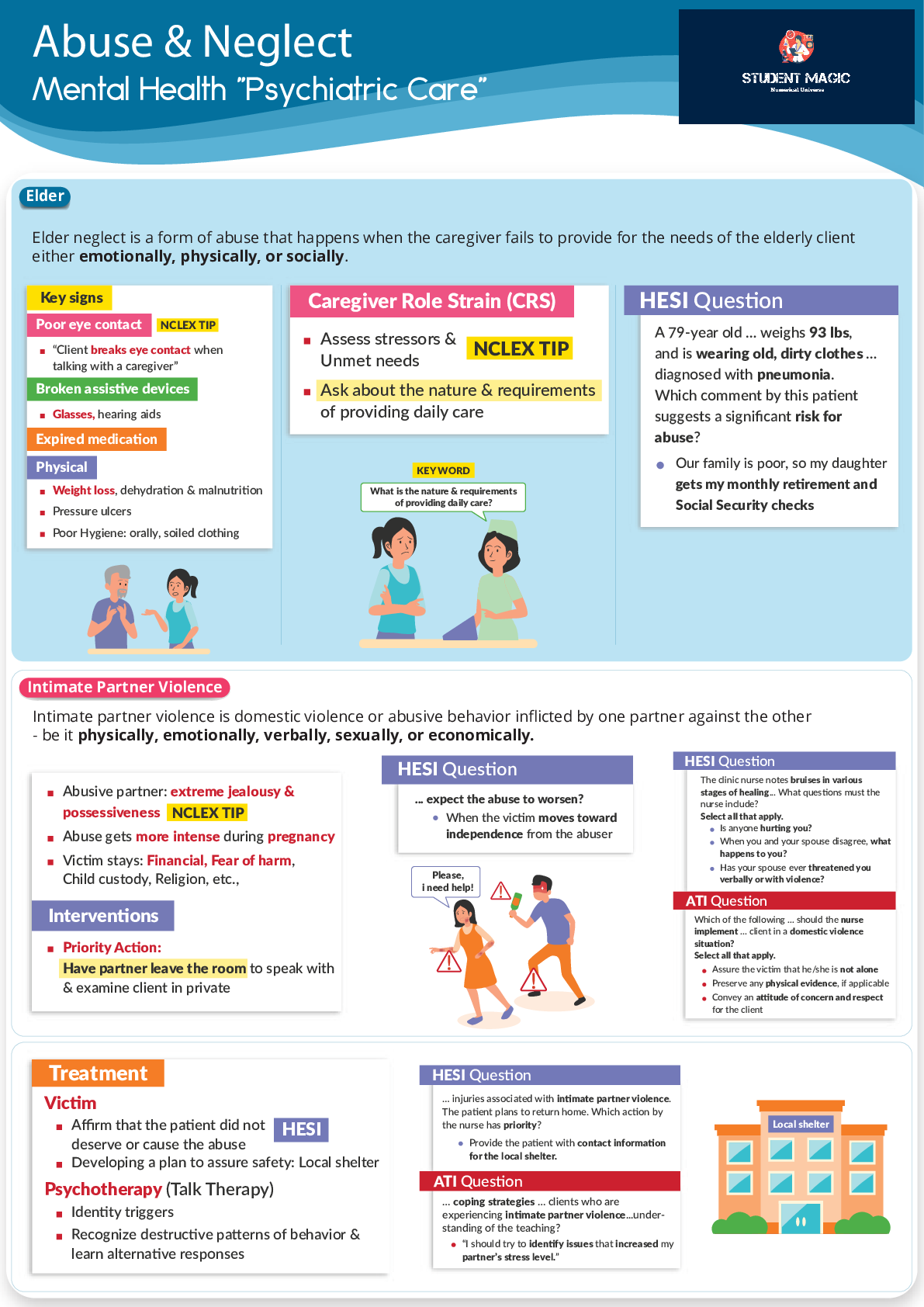
CHAPTER 5 Bound States: Simple Cases 5.1 Unless the confines of the particle are small, compared to its wavelength, it will not behave as a wave. And unless it is bound, it will not form standing w... aves, which are the basis of quantization. 5.2 The electron in an enclosure so small as an atom behaves as a standing wave, which gives it quantized properties. In quantum mechanics, these standing waves correspond to charge densities that are static in time, so they need not emit electromagnetic radiation. 5.3 For each force there is a potential energy. F = – dU/dx. One follows unambiguously from the other. They are not independent ideas, so it is reasonable to use a single term for both. 5.4 A charged particle would not be restricted to only certain energies. But the electrons orbiting atoms behave as standing waves, which are inherently quantized. They have only certain allowed energies, so the energy they emit in the form of light can take on only certain values. In an incandescent bulb, high speed thermal motion of charges produces the light, but this motion is essentially continuous. 5.5 In a stationary state, it is not a particle, but a wave, and the thing that is stationary is the probability density. It does not change with time, nor, if the particle is charged, does the charge density. 5.6 It never is. Were it zero, the probability of finding the particle would at least temporarily disappear. This is not allowed. 5.7 The sine in the wave function goes to zero identically. We cannot have zero probability of finding the particle. 5.8 As the walls are moved closer, the wavelengths of all standing waves would be made shorter, implying larger momentum and thus kinetic energy. As the squeezing continues, the kinetic energies of one state after another will exceed the height of the potential energy walls, whereupon the particle would no longer be bound. 5.9 5.10 Its wave function will penetrate less into the classically forbidden region, which in turn will reduce its wavelength and thus slightly increase its energy. 55Chapter 5 Bound States: Simple Cases 5.11 (b) The outer wall is of height zero, so the particle can be bound only so long as its total energy E is negative. (a), (c) and (d) are on sketch below. 5.12 The second and fourth have nodes in the middle, and simply assume a longer wavelength as the wells separate. A larger wavelength implies a smaller momentum and thus kinetic energy. (b) This first and third are required to die off in the classically forbidden region, where they would naturally have an antinode. Thus, they assume somewhat tortuous but effectively shorter wavelengths than in one single well. (c) Something approaching an antinode can form in the middle, giving a correspondingly long wavelength and low energy for the ground state. 5.13 It tends to get longer near the extreme edges. The reason is that the kinetic energy is low there, so the momentum is small, corresponding to a large wavelength. 5.14 All form standing waves and have a ground state that is not of zero (kinetic) energy. The infinite well and harmonic oscillator “hold” an infinite number of states, while the number is limited for the finite well. In the infinite well, wave functions do not extend outside the walls, but for the others, they do. The energies in the infinite well get farther apart; for the oscillator they are equally spaced. The spacing of levels in the finite well is less easily characterized. 5.15 Its wavelength could not be constant. Since E is constant, the kinetic energy increases on the left, meaning a larger momentum and a smaller wavelength there. 5.16 For the harmonic oscillator, the “walls” get farther apart as the energy increases, and the result is equal spacing of levels. The higher levels in the infinite well are comparatively more closely confined, so they would have shorter wavelength, higher momentum and would thus have higher and higher energy relative to the oscillator. (b) If higher energy levels are actually closer together, it must be that the “walls” get farther apart with increasing energy even faster than in the harmonic oscillator. 5.17 For the infinite well, there is no maximum energy, so no minimum photon wavelength. There is a minimum energy difference; the n = 1 and n = 2 state are as close together as is possible, so a transition between them would produce the maximum possible wavelength photon. The oscillator is essentially the same, with no minimum wavelength, and with a maximum that would correspond to a transition between any two adjacent levels (equal spacing). The atom does have a maximum energy of zero, so the largest energy transition, from E = 0 to the lowest (negative energy) ground state would yield a minimum wavelength photon. There is no 5 [Show More]

Last updated: 1 year ago

Preview 1 out of 23 pages